Design of filters in intermediate frequency PCM/DPSK demodulator

　　I. Introduction

　　The IF PCM/DPSK telemetry signal fully softened demodulator uses software method to demodulate the digital signal directly sampled by IF. The result of intermediate frequency sampling greatly increases the amount of data, which puts pressure on subsequent digital signal processing. In order to solve this problem, in the fully soft demodulator, the high sampling rate signal after downconversion to baseband is decimated and filtered, and the amount of baseband signal data is reduced as much as possible while satisfying Nyquist's law, and then the decimated signal is The baseband signal is subjected to arithmetic processing. This article will study the design and softening implementation of digital cascade decimation filters in fully softened demodulators for intermediate frequency PCM/DPSK telemetry signals. 

　　2. Basic principles

　　Since the sampling rate of the input signal in narrowband digital IF processing is very high and the passband bandwidth and transmission band of the decimation filter are required to be very narrow, this will make the number of filter sections very large and the computing efficiency very low. Therefore, in order to effectively achieve sampling rate conversion, this article will adopt a multi-stage extraction method. Since the filter is decomposed into multiple stages and different forms of digital filters are used for extraction, the relative transition band of each stage of the filter is greatly increased, and the number of sections of each stage of the filter is greatly reduced, so it can be greatly reduced. The amount of filtering operations and the amount of storage of filter coefficients.

　　The digital cascade decimation filter in the intermediate frequency PCM/DPSK telemetry signal fully softened demodulator is divided into 3 levels according to the requirement of a decimation ratio of 10: the comb decimation filter realizes 5 times of signal extraction, and the half-band decimation filter realizes 2 times of signal extraction. And a general low-pass filter completes the compensation filtering. Its structural block diagram is shown in Figure 1. Among them, H1(z), H2(z) and H3(z) represent the frequency response of comb filter, half-band filter and general low-pass filter respectively, fs is the input sampling rate, f1=fs/5 is The output sampling rate after passing through the comb decimation filter, f2=fs/10. 

　　3. Filter design

　　The decimation filter is composed of a filter and a decimator, so no matter what form of decimation filter it is, it is actually a digital low-pass filter. Therefore, this article uses the equal ripple method to design all levels of FIR decimation filters and uses Matlab to complete it. The design of the cascade decimation filter is presented.

　　1. Comb Decimation Filter (CIC)

　　Since the sampled digital signal spectrum is a periodic continuation of the original analog signal spectrum according to the sampling rate, in order to ensure that the baseband spectrum does not alias during the signal extraction process, it is necessary to filter the part that may be mixed into the baseband spectrum. remove. In view of this consideration, a comb filter is used in the first stage of the multi-stage filter, and the transition band between the baseband and the stopband is filtered through the subsequent filter.

　　The CIC filter is a linear phase low-pass FIR filter, and its coefficients are all 1, which is relatively simple to implement. However, research shows that the stopband attenuation of the filter obtained in this way is small and cannot meet actual needs. In order to improve the stopband attenuation of the filter, the modified form of the CIC filter is used:

　　Figure 2 shows the frequency response diagram of the comb filter when N=5 and k=4. As can be seen from the figure, the frequency response has been significantly improved, its stopband attenuation reaches 80 dB, and the length of the filter is p=17. At this time, the frequency response H1(z) of the comb filter is

?

2. Half-band decimation filter

　　The half-band filter is a special linear phase filter. Nearly half of its coefficients are exactly zero, so the computational complexity of filtering is reduced by nearly half compared with other linear phase filters of the same length. . According to the frequency characteristics of the half-band filter and the advantages of less computational complexity, it can be used as a decimation or interpolation filter with a transformation factor of 2.

　　However, if the design method of the equal ripple filter is directly used to design the half-band filter, the condition that nearly half of the coefficients of the filter are zero cannot be utilized, and the filter design process will take a long time. Therefore, first use the equal ripple method to design a full-band linear phase filter prototype, and then design a half-band filter based on this prototype [3]. In the design, the passband side frequency ωp=(2e+6)Hz, the stopband side frequency ωc=(2.5e+6)Hz, the passband ripple δp=1 dB, and the order of g(n) is m=19 . Then according to the relationship of m=(N-1)/2, the order N=39 of the half-band filter is found. Finally, the coefficient h2 of the half-band filter is obtained as

　　The remaining even-numbered position coefficients are all zero.

　　Using the above method to design a half-band filter, we only used the cost of designing a filter with a length of (N-1)/2=(39-1)/2=19 to design a half-band filter with a length of N=39. , so for large N values, this greatly saves design time and obtains higher design accuracy. The frequency response of the half-band filter is shown in Figure 3.

　　3．General low pass filter

　　Although the CIC filter has low-pass characteristics, the passband is not flat, but has a certain roll-off, which distorts the signal spectrum. The wider the bandwidth of the signal, the more severe the distortion will be. Therefore, compensation is required with ordinary FIR filters. The frequency response of the CIC filter is H1(z), then the frequency response of the compensation filter in the passband is

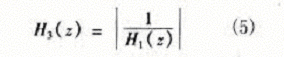
　　4. Conclusion

　　This paper uses the principle and method of cascade decimation filtering to realize the design of digital decimation filters in the demodulation of intermediate-frequency PCM/DPSK telemetry signals. It can be used for decimation filtering of digital signals under high sampling rates, advancing the sampling time from baseband to intermediate frequency. , reducing the front-end hardware circuit, reducing the complexity of the system, and improving the accuracy of the system. 

　　references

　　[1] Zhang Jian, Xiang Jingcheng. Introduction to Software Radio Technology [M]. Chengdu: University of Electronic Science and Technology of China Press, 2001.

　　[2] Yang Xiaoniu. Principles and Applications of Software Radio [M]. Beijing: Electronic Industry Press, 2000

　　[3] Liu Yicheng, Luo Weibing. Signal processing oversampling converter [M]. Beijing: Electronic Industry Press, 1997