Steady-state calculation of non-sinusoidal periodic signal circuit

For steady-state circuits excited by non-sinusoidal periodic signals, it is impossible to use the calculation methods of DC circuits or sinusoidal AC circuits for analysis and calculation. Instead, the non-sinusoidal periodic signal excitation must first be decomposed into the sum of sinusoidal components of different frequencies using Fourier series, and then the circuit response under the excitation of each frequency component is calculated separately. Finally, the superposition theorem is used to superimpose the response components to obtain the steady-state response. The main steps of the calculation process can be divided into three steps:

(1) Decompose the given non-sinusoidal periodic excitation source into a Fourier series expression, that is, decompose it into the sum of the DC component and each harmonic component, and determine the required number of harmonic terms based on the convergence of the expansion terms and the required accuracy;

(2) Calculate the circuit response under the excitation of the DC component and the harmonic components of each frequency respectively. The DC component uses the DC circuit analysis method, in which the inductor is short-circuited and the capacitor is open-circuited; for sinusoidal components of different frequencies, the sinusoidal circuit phasor analysis calculation method is used. At this time, it should be noted that the impedance of the circuit changes with the frequency. When calculating each component separately, a corresponding circuit diagram should be made;

(3) Apply the superposition theorem to add the harmonic components of the output response to obtain the total response value. Note that each harmonic response should be expressed as an instantaneous form in the time domain before superposition (because the addition of phasor forms of different frequencies is meaningless).

The following specific examples are used to illustrate the periodic non-sinusoidal steady-state analysis of linear circuits.

Example 6-2-1 The circuit is shown in Figure 6-2-1. Given , , , power supply voltage , fundamental angular frequency , find the current flowing through the resistor and the voltage across the inductor .

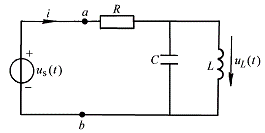
Figure 6-2-1

Solution: The excitation voltage source in this problem has been decomposed into harmonic components, so the calculation of each harmonic can be performed directly. For the calculation of the DC component, the method of solving the problem of a general DC circuit can be used to draw the corresponding DC circuit as shown in Figure 6-2-2a. Given that

For the fundamental wave component , the corresponding circuit is shown in Figure 6-2-2b, and the input impedance of ab terminal is:

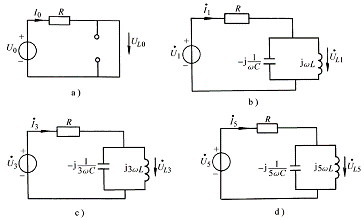
Figure 6-2-2

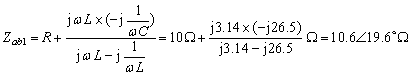
Voltage across the inductor:

That is:

,

For the third harmonic component, its equivalent circuit is shown in Figure 6-2-2c, and its input impedance is:

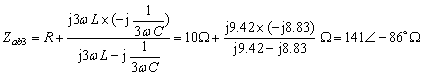
Voltage across the inductor:

That is:

,

For the fifth harmonic, the equivalent circuit is shown in Figure 6-2-2d, where the input impedance of the ab terminal is:

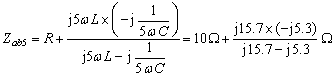
Voltage across the inductor:

That is:

,

Finally, the current flowing through the resistor is:

From the calculation results, it can be seen that the circuit presents different characteristics for components of different frequencies. When the third harmonic is excited, the input impedance is particularly large, so the current component generated is small, which is due to the proximity to the resonant frequency point of the circuit.

The following discusses the effective value and power of non-sinusoidal periodic signals. The effective value of a periodic signal has been defined as:

For non-sinusoidal periodic signal current , it can be expanded into Fourier series:

Substituting valid value expressions are:

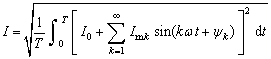
Expanding the square inside the square root sign gives two types of expressions. One type is the square of the current component with the same frequency, which can be calculated as:

The second type is the product of currents of different frequencies. From the orthogonality of trigonometric functions, we know that the product of two sine functions of different frequencies is zero when integrated on , that is:

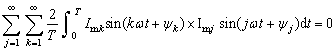
So the effective value of the periodic non-sinusoidal alternating current is:

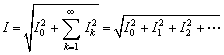(6-2-1)

In the formula, Ik is the effective value of each harmonic. Similarly, the effective value of non-sinusoidal periodic voltage can be deduced as _:

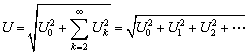(6-2-2)

Power of a non-sinusoidal periodic signal:

,

In the formula,

,

The average power is:

Substituting the expanded form of and into, the expression of the product is composed of the product of the sine quantity of the same frequency and the sine quantity of different frequencies. Considering the orthogonality of trigonometric functions on , it can be deduced that:

(6-2-3)

In the formula, is the phase difference between the kth harmonic voltage and current. It can be seen from the formula that the average power of the non-sinusoidal signal is equal to the sum of the average power of each harmonic signal.