Research on chaotic synchronization circuit based on analog inductance

As we all know, the modern trend in electronics is to reduce the size of circuits, and it is relatively simple to reduce the size of resistors and capacitors in integrated circuits. As for passive inductors, they are bulky and are not conducive to integration. This is because there is no electromagnetic effect in the semiconductor, and the semiconductor is the main material of the integrated circuit. Therefore, the magnetic material that makes up the iron core and the wires that make up the inductor winding must be deposited on the surface of the semiconductor. This structure can only get very low The amount of inductance; in addition, the size of the inductor has a great relationship with the quality factor. The smaller the size, the smaller the quality factor, so small inductors usually cannot be applied. For the above reasons, in order to eliminate inductance in a circuit, active devices can be used to simulate the inductance. The so-called analog inductor is to replace each inductor in the circuit with a comprehensive circuit. This theory enables the inductive components to be miniaturized, chip-shaped and integrated in the circuit. This article applies active inductors to chaotic circuits and conducts simulations to obtain ideal results.

1 Chaos and Chaos Synchronization Principle

The so-called chaos refers to the random-like output generated by a deterministic system. The so-called deterministic circuit means that the parameters and inputs of the circuit are certain values ​​and there are no random factors. The so-called uncertainty,

Random-like output means that the output of the circuit is neither periodic nor quasi-periodic; it neither tends to infinity nor to stationary, but presents an output that never repeats within a certain area. Generally speaking, chaotic synchronization belongs to the category of chaos control. Several types of chaotic synchronization have been discovered so far, one of which is the synchronization scheme proposed by Pceora and Carroll. There is a relationship between driving and being driven in the circuit of this solution. The driving circuit can be divided into a stable part and an unstable part. The stable part copies a response, and then the response system and the driving system are coupled with the driving signal. This can Achieve synchronization between the corresponding system and the drive system.

With the deepening of research on nonlinear circuits, there have been many reports of actual circuits that generate chaos and are used to study the mechanism of chaos generation. Chaos phenomena widely exist in nonlinear circuits. A typical circuit that has been studied in depth is Chua's circuit. Chua's circuit is shown in Figure 1(a). The nonlinearity in the circuit is introduced by a piecewise linear negative resistor. The volt-ampere characteristics of the nonlinear resistor are shown in Figure 1(b).

When the parameters of the circuit meet certain conditions, a self-oscillating attractor that becomes a double scroll will be generated. Figure 2 is a Chua's chaotic synchronization circuit.

2 Analog Inductor

This article introduces three commonly used analog inductor circuits: Riordan inductor circuit, lossless analog inductor circuit and low-loss analog inductor circuit.

2.1 Riordan inductor circuit

This circuit (Figure 3) is composed of 2 integrated operational amplifiers, 4 resistors and 1 capacitor. Since the operational amplifier is regarded as an ideal integrated operational amplifier, the open-loop differential mode voltage amplification factor Aod≦∞, the current flowing into the two input terminals I+≦I_≦0, U+≦U_.

The first op amp implements a non-inverting proportional arithmetic circuit, so we can get:

Therefore, the Riordan circuit can be equivalent to an analog inductor with L=R2C.

2.2 New lossless analog inductor circuit

This circuit (Figure 4) consists of 1 op amp, 4 resistors and 2 capacitors. Ui is the input signal and Uo is the output signal. From the characteristics of the ideal operational amplifier, it can be concluded that:

Therefore, this circuit can be equivalent to an analog inductor with L=2R2C.

2.3 Low-loss analog inductor circuit

This circuit (Figure 5) is composed of 1 op amp, 4 resistors and 1 capacitor. Ui is the input signal and Uo is the output signal.

According to the characteristics of the ideal operational amplifier, it can be listed:　

It can be concluded that this circuit can be equivalent to a series combination of a resistor and a L=R1R2C.

3 Simulation research

Replace the inductors in Figure 2 with the above three simulated inductors for simulation. Observe the time domain waveforms, chaotic attractors and output voltage spectra of the three circuits when 18mH simulated inductors are used instead of actual inductors.

3.1 Application of Riordan circuit in chaotic synchronization circuit

Taking the parameters in the Riordan circuit introduced earlier as R=1kΩ and the capacitor C=18nF, the Riordan circuit can be equivalent to an inductor of L=R2C=(103)2×18×10-9=18mH.

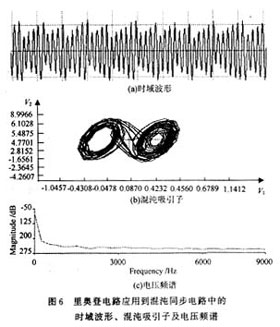
The time domain waveform, chaotic attractor and voltage spectrum obtained by applying it to the chaotic synchronous circuit are shown in Figure 6.

3.2 Application of new lossless analog inductance circuits in chaotic synchronization circuits

Taking the parameters in the lossless analog inductor circuit introduced earlier as R=1kΩ and C=9nF, this new analog inductor circuit can be equivalent to a L=2R2C=2×(103)2×9×10-9=18mH inductance.

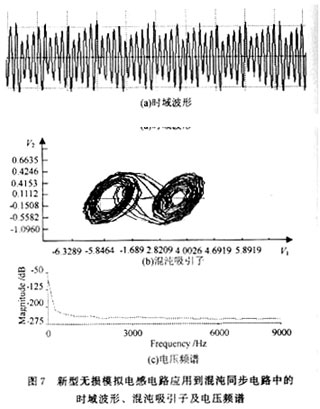
The time domain waveform, chaotic attractor and voltage spectrum obtained by applying it to the chaotic synchronous circuit are shown in Figure 7.

3.3 Low-loss analog inductor circuit applied to chaotic synchronization circuit

Taking the parameters in the low-loss analog inductor circuit introduced earlier as R4=R2=0.05kΩ, R1=R3=4kΩ, and C=90nF, the low-loss analog inductor circuit can be equivalent to a L=R1R2C=4×103×0.05 ×103×90×10-9=18mH inductance.

The time domain waveform, chaotic attractor and voltage spectrum obtained by applying it to the chaotic synchronization circuit are shown in Figure 8.

4 Conclusion

By applying the Riordan circuit, the new lossless analog inductor circuit and the low-loss analog inductor circuit to the chaotic synchronous circuit for simulation research, it is found that the analog inductor can replace the actual inductor without affecting the chaotic characteristics of the chaotic circuit. Based on the analog inductor The chaotic circuit not only has the spectral characteristics of white noise, but also the autocorrelation function has a behavior close to the delta function, which provides conditions for the development and research of integrated functional chaotic oscillators. The simulation results show that the chaotic circuit based on analog inductors not only does not affect the characteristics of the chaotic circuit itself, but also has the characteristics of small size and easy integration. Therefore, it has very broad application prospects.