Harmonic and reactive current detection based on instantaneous reactive power theory

1 Introduction

After the 1980s, power electronics technology developed rapidly. The application of various frequency converters, converters, switching power supplies and reactors has increased day by day. The resulting harmonic pollution has also become increasingly serious, causing serious distortion of voltage and current in the power system. It affects the normal operation of instruments and meters, increases the loss of power devices, and endangers the safe operation of the power system. At present, harmonic pollution has become one of the serious public hazards of the power system. It is particularly urgent to solve the harmonic problem of the power system. The harmonic problem of the power system involves a wide range of aspects, including harmonic detection, harmonic analysis, harmonic source analysis, power grid harmonic tide calculation, harmonic suppression, harmonic standards, etc. Harmonic detection is an important branch of harmonic problems and the basis for solving other harmonic problems. Since the harmonics of the power system are affected by factors such as randomness, distribution, and non-stationarity, it is not easy to accurately detect them. With the widespread application of power electronic devices, harmonic and reactive power problems have become a hot topic of research. In 1983, Japanese scholar Yasufumi Akagi proposed the instantaneous reactive power theory of three-phase circuits, also known as the pq theory, which provided a new method for harmonic and reactive power detection in three-phase circuits.

2 Three-phase instantaneous reactive power theory based on ip-iq

In order to overcome the shortcomings of the pq theory detection affected by voltage quality, the pq theory has been continuously developed and improved to form a three-phase instantaneous reactive power theory based on ip-iq. The core idea of ​​this theory is to transform the three-phase current that satisfies ia+ib+ic=0 through Park transformation without zero-sequence components to obtain ip and iq. That is:

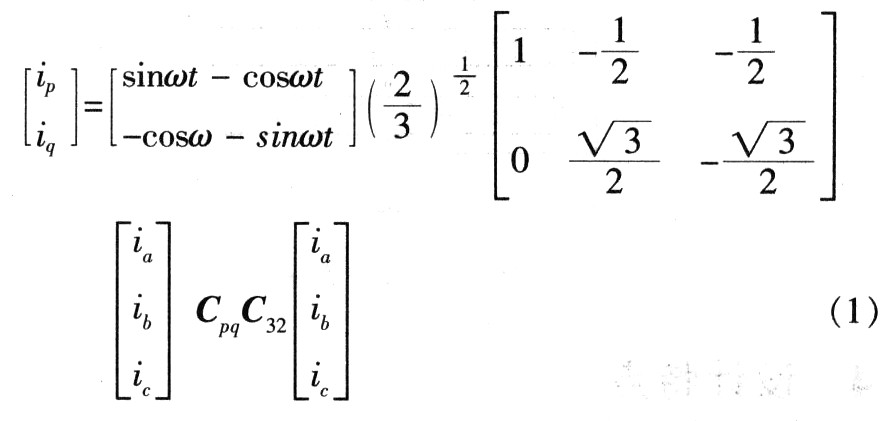
It can be seen that the current detection at this time is only related to the electrical angle ωt of the A phase voltage, and the distortion of the voltage wave has no effect on the detection result. Among them, C32 is the coordinate transformation matrix from three phases to two phases; Cpq is the rotation coordinate transformation matrix. When the three-phase current is symmetrical, the detected current is:

Where k is an integer, ω is the angular frequency, Ikm and θk are the amplitude and initial phase of each current. Substituting (2) into (1) yields the following equation:

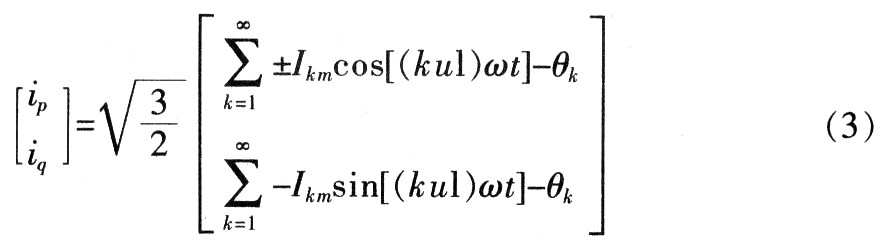
When k=1, 7, 13..., the sign is taken as upper; when k=5, 11, 17..., the sign is taken as lower. If the AC component in equation (3) is filtered out, we can get:

From formula (4), we can see that the active current and reactive current of the fundamental component in the traditional sense correspond to each other. Because ip and iq can be separated by LPF to obtain the DC component , if is reversely transformed at the same time, the fundamental components iaf, ibf, and icf can be obtained as follows:

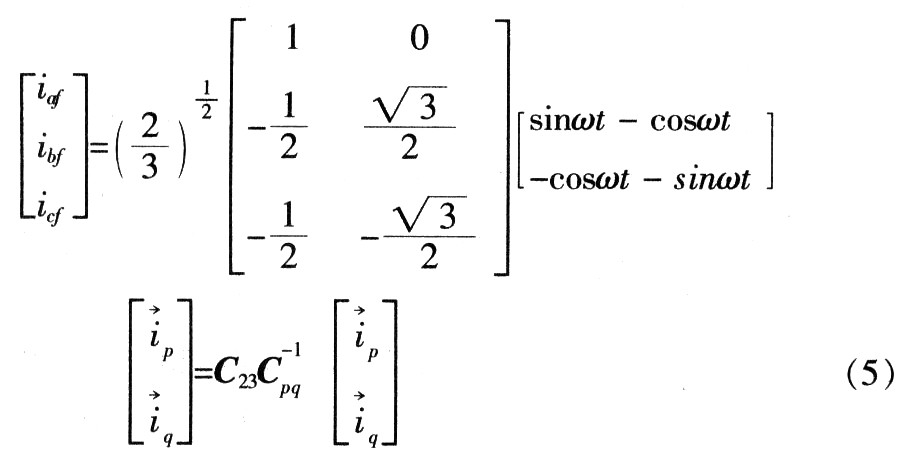
Where C23 is the coordinate transformation matrix from two phases to three phases. Then the harmonic currents iah, ibh, ich can be obtained as:

Further analysis of the harmonic currents iah, ibh, and ich can reveal the specific conditions of the higher harmonics contained in the three-phase alternating current, thereby enabling real-time detection of the higher harmonics and targeted filtering control.

If only iq is transformed inversely according to equation (1), the instantaneous values ​​of reactive current components iaq, ibq, icq are obtained as follows:

Formula (7) can separate the instantaneous reactive current in the three-phase AC power without delay. In practice, the control system can be designed according to the current value to achieve rapid reactive compensation. It can be seen that the introduction of the theory of instantaneous reactive power of three-phase circuits provides a theoretical basis for the rapid detection of reactive current and high-order harmonic current.

3 Harmonic and reactive current detection based on reactive power theory

After the three-phase instantaneous reactive power theory was proposed, it was soon used to detect harmonics and reactive current in power systems due to its real-time performance in detecting current. With the further development of the theory, various detection methods were derived. Among them, the representative ones are: p-q detection method and ip-iq detection method. The following discusses the p-q detection method:

This method calculates p-g according to the definition, when the voltage wave is undistorted (i.e. a standard sine wave), and the current contains reactive and harmonic components:

Where k is an integer representing the harmonic order, ω is the angular frequency; Ikm and ψk are the amplitude and initial phase of each current.

Transform (8) into the αβ coordinate system and substitute it into (5) to obtain:

In the formula, n=3k+1. When n=3k+1, the upper sign is taken in the calculation formula (9); when n=3k-1, the lower sign is taken in the calculation formula (9). It can be seen that formula (9) can be divided into two parts, the first half of which is the DC component corresponding to n=1, that is, the fundamental active power and fundamental reactive power in the power. The second half is the power of the AC part corresponding to n>1, that is, the higher harmonic active power and reactive power in the power. The calculation block diagram when using this detection method to detect harmonics is shown in Figure 1.

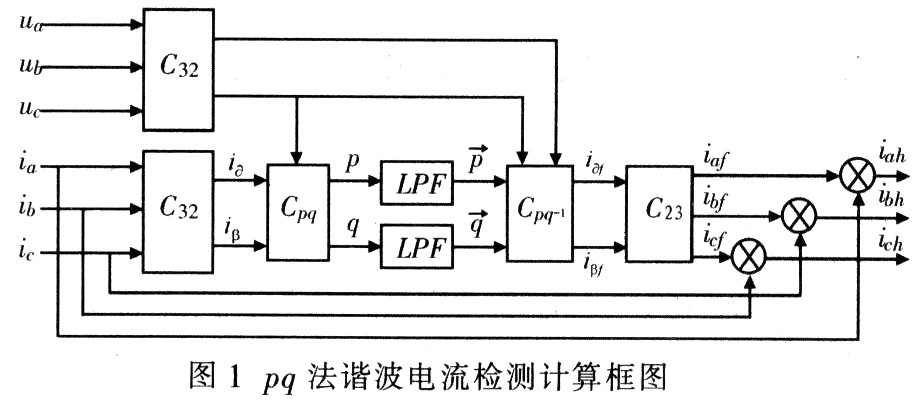
The detection method is briefly described as follows: first, the sampled three-phase voltage and current values ​​ua, ub, uc and ia, ib, ic are transformed by αβ to obtain uα, uβ and iα, iβ; then p, q are calculated by definition; the DC components p, q in p, q are obtained by low-pass filter; p, q are mutually inversely transformed to obtain the fundamental current components iaf, ibf, icf in the three-phase current. Subtract the fundamental current components iaf, ibf, icf from the three-phase current ia, ib, ic to obtain the higher harmonic components iah, ibh, ich in the current. When the grid voltage is not distorted, it can be seen from the above derivation that the detection of the fundamental current components iaf, ibf, icf is accurate, and thus the detection values ​​of the higher harmonic components iah, ibh, ich are also accurate. The following discusses the detection situation when the grid voltage is distorted.

Assume the grid voltage is:

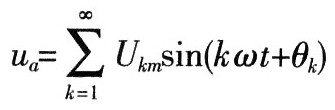
It can be seen from the above formula that the DC components p and q contain not only the fundamental active power (reactive power) but also the active power (reactive power) generated by higher harmonics. Therefore, the fundamental current components iaf, ibf, and icf in the three-phase current calculated by this formula must have errors, which will inevitably cause errors in the detected higher harmonic components iah, ibht, and ich. This is also the biggest shortcoming of the pq detection algorithm. To detect the sum of harmonic current and reactive current for comprehensive compensation, the detection block diagram in Figure 2 can be used.

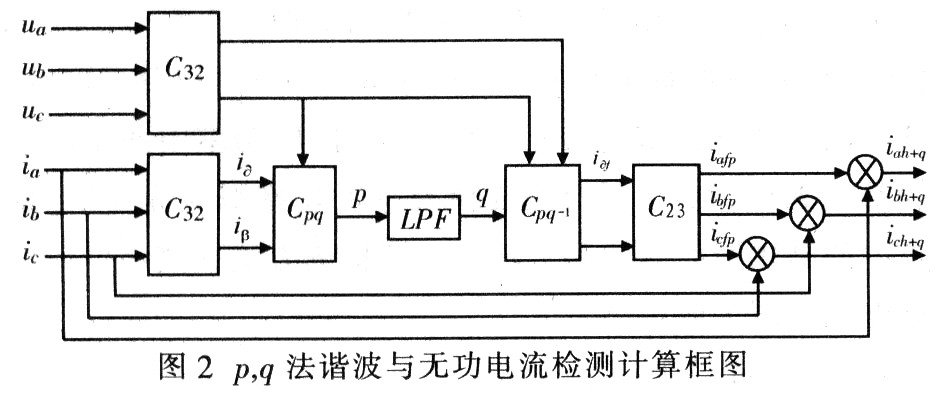
In this detection block diagram, only p is low-pass filtered, while q is not filtered. p and q are inversely transformed to obtain the fundamental current components iaf, ibf, and icf in the three-phase current. At this time, iaf, ibf, and icf only contain the fundamental active current component; the fundamental current component is subtracted from the three-phase currents ia, ib, and ic, and iaf, ibf, and icf are used to obtain the sum currents iah+q, ibh+q, and ich+q of the higher harmonics and reactive currents in the current.

4 Conclusion

Accurate and real-time detection of harmonic currents and reactive currents in the power grid is the key to suppressing harmonics and reactive compensation. Based on the instantaneous reactive power theory, the three-phase harmonic currents were detected. The feasibility and effectiveness of the harmonic detection method based on the instantaneous reactive power theory were proved, providing accurate and real-time harmonic and reactive components for suppressing harmonics and reactive compensation.