1 Overview
Radio engineers strive to find an electromagnetic wave radiated by a radio fuse that can radiate freely in all directions without any reflection interference. In other words, they strive to find a free space that can provide a radio fuse to work under laboratory conditions.
The emergence of echo-free absorption chambers meets the needs of this type of engineering research. This type of echo-free absorption chamber refers to a room whose inner walls are made of radio wave absorbing materials. This special wall allows the incident electromagnetic waves to be absorbed to the maximum extent and reflected to the minimum extent, and a certain part of the room can form an echo-free area close to the "free space condition".
The use of anechoic absorption chamber is quite extensive. Almost all radio parameter tests can be carried out in the anechoic absorption chamber, such as antenna characteristic test, radar cross section test, whole system sensitivity test and other simulation tests. The radio equipment on various aircraft, missiles and artificial satellites can also be comprehensively tested in the anechoic absorption chamber.
2 Performance analysis of absorbent materials
As the name implies, echo-free absorbing materials are used to absorb electromagnetic wave energy, just like black pigment absorbs light. Under normal circumstances, to produce a significant absorption effect, the absorber needs to be at least 1/4 wavelength thick.
The absorption performance of an absorber can be understood in this way: when an electromagnetic wave hits the surface of an object, part of the energy of the electromagnetic wave will be reflected back, and part of the energy will pass through the boundary to the second medium. These electromagnetic waves that enter the surface of the material penetrate the medium through various channels and become radiation energy on the other side of the surface of the material, and the intensity of the electromagnetic wave becomes weaker; and the part of the electromagnetic wave energy that has not penetrated is absorbed. Therefore, in order to achieve ideal absorption of electromagnetic waves, first, the absorbing material should be able to allow the electromagnetic waves incident on the surface of the material to penetrate as much as possible, that is, to enter the absorbing material, so that the reflection of the electromagnetic wave is minimized. Secondly, if the electromagnetic wave is to enter the absorbing material completely, the electromagnetic wave incident line must be perpendicular to the surface of the absorbing material. Thirdly, the electromagnetic wave energy that penetrates into the absorbing material should be able to effectively absorb all the incident electromagnetic energy. It can be seen from this that the performance of the darkroom (such as the size of the reflectivity level) depends critically on the absorbing material used. The absorbing material usually used is a pyramid, and its shape is shown in Figure (1):
If a pyramid-shaped absorbing material is used, no matter what polarization (parallel polarization or vertical polarization) the electromagnetic wave is incident on the absorbing material, there are always two faces in the oblique incident state of parallel polarization, and the other two faces in the vertical polarization state. In the case of oblique incidence, the interface reflection of vertical polarization is generally greater than that of parallel polarization.
In the case where both polarizations are present, the overall reflection coefficient is between the reflection coefficients of only parallel polarization and perpendicular polarization, and is closer to the perpendicular polarization.
According to the relevant literature [1], the characteristic impedance of electromagnetic wave reflection on the interface under oblique incidence is:
Where θi is the incident angle, θt is the refraction angle of the wave in the medium, η is the characteristic impedance of the incident medium, and η0 is the characteristic impedance of air. In general, the characteristic impedance of air is the characteristic impedance of the incident medium.
The interface reflection coefficients of two polarized electromagnetic waves at different incident angles are shown in Figure (2), assuming an absorbing material.
It can be seen that the interface reflection coefficient of the vertically polarized wave always increases with the increase of the incident angle. However, the interface reflection coefficient of the parallel polarized wave first decreases with the increase of the incident angle, and can be much smaller than that of the vertical incidence. Then it increases with the increase of the incident angle. Except for the case of small incident angle and incident angle close to 90°, in most cases, the interface reflection of the parallel polarized wave is much smaller than that of the vertically polarized wave.
3 Analysis of the Quiet Zone of a Small Cylindrical Microwave Dark Box
Generally speaking, the electrical performance of a darkroom is mainly demonstrated by the characteristics of the quiet zone, which are expressed in terms of the size of the quiet zone, the reflectivity level within the quiet zone, the cross-polarization, the field uniformity, the path loss, the operating frequency range and the inherent radar cross-section parameters.
The so-called quiet zone refers to the area in the darkroom that is least disturbed by various stray waves. Its size is related not only to the geometric shape of the darkroom, the operating frequency, and the electrical properties of the absorbing material, but also to the required reflectivity level, the shape of the quiet zone, and the structure of the darkroom. For a small cylindrical darkroom, due to its symmetrical structure and the same absorbing material laid on the inner wall, the quiet zone is cylindrical, with the axis consistent with the axis of the darkroom, and its diameter satisfies the following formula:
Where: λ--wavelength, R--distance between the transmitting and receiving antennas. The reflectivity level in the quiet zone can be described by the following formula:
Where: ED is the incident field in the axis direction of the small cylindrical dark box;
ER is the equivalent reflected field synthesized by reflection, diffraction and scattering at the measurement point.
The reflectivity level at any point in the small cylindrical dark box changes with the operating frequency. In order to accurately detect the reflectivity level, the following points must be clarified first:
(1) Move the receiving antenna longitudinally (along the cylindrical axis). If the received signal strength changes with 1/R [2] (R is the distance between the transmitting and receiving antennas), it indicates that the cylindrical dark box test meets the far-field measurement conditions. If the received signal oscillates with a period of half a wavelength, it indicates that the coupling between the antennas is strong. If the oscillation period is greater than λ/2, it indicates that there are multipath reflection signals.
(2) At the end face of the point to be tested, move up and down, left and right, or longitudinally along the axis to detect whether the field strength is uniform. If the receiving level fluctuation is better than 0.25dB and is b
asically symmetrical up and down, it indicates that the incident field tapering amplitude requirements are met.
4 Using the space standing wave method to test the reflectivity level of a small cylindrical microwave dark box
Physically speaking, the non-directional probe antenna is moved horizontally or vertically along the axis of the dark box to measure the spatial standing wave curve. The reflectivity level is determined based on the maximum and minimum values Emax (dB) and Emin (dB) of the standing wave curve [3], as shown in Figure (3) and Figure (4):
However, the actual antenna is directional, so formula (6) must be corrected. That is, the incident field received by the antenna at angle φ is lower than the incident field when φ=0° by AdB. In the φ direction, the difference in dB between the maximum and minimum values of the standing wave curve is:
Then the reflectivity level in the φ direction is:
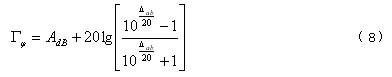
Figures (3) and (4) respectively show the standing waves and standing wave curves formed by the incident and reflected waves in free space. Obviously, the actual physical meaning of this method is: under ideal conditions, there are only direct waves in the dark box, and most of the electromagnetic wave energy projected onto the absorbing material is absorbed. However, when there are certain stray waves (such as reflected waves, diffracted waves, scattered waves, etc.), these coherent beams form peaks when the phase difference between the two waves is 2nπ (n=1, 2, 3) under the same polarization conditions. Where the phase difference is 2(n+1)π, the two waves cancel or partially cancel to form nodes. Many peaks and nodes appear in the cylindrical dark box, forming a spatial standing wave distribution with a very complex field structure. Therefore, the reflectivity level in the quiet zone is much smaller than the reflectivity level in other spaces.
5 Main methods for determining the reflectivity level of a small cylindrical microwave dark box
(1) Measure the radiation pattern of the receiving antenna and mark the corresponding radiation pattern level A1 (dB) at the required azimuth φ.
(2) Point the antenna's maximum radiation direction to the angle φ, move the antenna horizontally and record the spatial interference wave curve at this time, as shown in Figure (5):
(3) Draw the envelope of the standing wave curve, calculate Δab from the maximum and minimum values of the envelope, and calculate its average level A1 (dB). If ED>ER, A1 (dB) is the directional pattern level of the receiving antenna. As shown in Figure (6):
(4) Repeat steps (2) and (3) at different angles to obtain a series of spatial standing wave curves. Then, the Δab value can be obtained from the envelope of the standing wave curve. When ED>ER, the reflectivity level in different directions can be obtained by equation (8). If ED>ER, the reflectivity level needs to be calculated as follows:
Since ED changes regularly with the movement of the antenna and ER changes irregularly, at a certain orientation angle, if the average value of the measured spatial standing wave curve changes irregularly, it can be determined that ER>ED. Alternatively, at this orientation angle, assuming that the average level of the standing wave curve is higher than the level of the radiation pattern at this orientation angle, it can also be determined that ER>ED.
6 Establishment of the test system and test steps for a small cylindrical microwave darkroom
(1) Establishment of test equipment
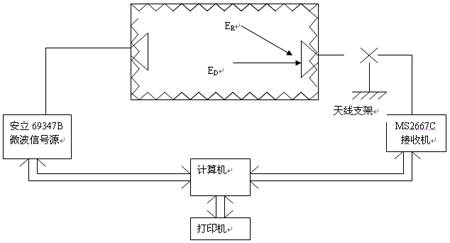
The test system for the reflectivity level of a microwave dark box mainly consists of a transmitting signal source (69347B), a receiver (MS2667C spectrum analyzer), a computer, a receiving antenna and a test bracket, see block diagram (7). The signal source is a transmitting antenna that outputs a microwave direct signal. The spectrum analyzer receives the reflected and direct signals from all directions, and the computer reads them out to draw a spatial standing wave curve and calculate the reflectivity level. The antenna bracket is used to control the vertical, left and right linear movement of the test antenna and the change of the angular posture. In order to accurately draw the spatial travel standing wave curve, the travel distance of the antenna must be greater than or equal to two wavelengths. Finally, by changing the orientation angle of the receiving antenna, a number of standing wave curves are obtained, thereby achieving the purpose of testing the reflectivity level of a small cylindrical microwave dark box.
(2) Testing process
According to the characteristics of the "VSWR" method, we installed the receiving antenna on the test bracket, placed the antenna on the central axis of the dark box and 15 cm away from the sharp wedge on the back wall, and changed the angle between the antenna and the central axis to test the reflectivity level.
(3) Determination of antenna test status
Here we only choose the vertical polarization state for testing. Secondly, considering the limitations of the tested box, only one point is selected at the test position, that is, the receiving antenna is 15cm away from the back wall wedge, and the pitch angle is ±20°, ±35°, and the receiving antenna moves up and down 2-4 wavelengths. Since the dark box is a cylinder, the reflection performance of any point on the inner wall of the dark box can be tested by rotating the dark box, while the reflection performance of the back wall of the dark box cannot be measured due to the limitations of the test conditions, so it is not considered for the time being.
(4) Z-direction (vertical) test
The Z-direction test is to move the receiving antenna up and down vertically with the axis of the box at a distance of 15 cm from the sharp wedge of the rear wall. The height of the up and down movement is ±3λ (±9 cm). The orientation angle of the antenna is ±20° and ±35°. Several interference curves are measured at these points. The vertical test is mainly to measure the reflection of electromagnetic waves by the upper and lower sharp wedges of the dark box.
At 0°, the curve (value) obtained by the receiving antenna is the uniformity of the field amplitude in the Z direction. Through the curve measured by the spectrum analyzer, the maximum level change value (Δab) and the average value of the curve (Ai) are calculated to obtain the directional pattern level value of the antenna at the orientation angle θ.
(5) Rotate the microwave darkbox to the required angle φi, repeat steps (3) and (4), and measure the reflectivity level of the remaining inner walls of the darkbox. The following is our test of the darkbox numbered 6CH-6W2 of the 802 Institute using the above method. The test data are as follows:
Table (1): Changes in the level when the receiving antenna moves up and down Test frequency: 10.125 GHz, the distance of 5 cm from the receiving antenna to the center axis of the dark box is defined as the starting position of the movement, and the receiving antenna takes the elevation angles of +20° and -20° respectively.
|
Moving distance (
mm
)
|
Level change
at
elevation angle
+20
° (
dB
)
|
Level change
at
-20
°
pitch angle
(
dB
)
|
|
0
|
61.09
|
61.24
|
|
2
|
61.08
|
61.22
|
|
4
|
61.08
|
61.17
|
|
6
|
61.04
|
61.17
|
|
8
|
60.98
|
61.12
|
|
10
|
60.96
|
61.08
|
|
12
|
60.95
|
61.06
|
|
14
|
60.91
|
61.04
|
|
16
|
60.96
|
61.03
|
|
18
|
60.97
|
61.03
|
|
20
|
60.97
|
61.03
|
|
22
|
61.00
|
61.04
|
|
24
|
61.00
|
61.10
|
|
26
|
60.98
|
61.15
|
|
28
|
60.99
|
61.17
|
|
30
|
60.96
|
61.19
|
|
32
|
60.94
|
61.28
|
|
34
|
60.89
|
61.37
|
|
36
|
60.88
|
61.31
|
|
38
|
60.86
|
61.42
|
|
40
|
60.86
|
61.47
|
|
42
|
60.83
|
61.53
|
|
46
|
60.80
|
61.58
|
|
48
|
60.74
|
61.63
|
|
50
|
60.71
|
61.65
|
|
52
|
60.66
|
61.72
|
|
54
|
60.90
|
61.74
|
|
56
|
60.89
|
61.74
|
|
58
|
60.79
|
61.74
|
|
60
|
60.46
|
61.69
|
|
62
|
60.45
|
61.67
|
|
64
|
60.73
|
61.63
|
|
66
|
60.72
|
61.58
|
Table (2): Total reflectivity level at different orientation angles
|
Azimuth
|
+20
°
|
-20
°
|
|
Directional pattern level value A(dB)
|
10
|
10
|
|
Maximum level change value Δ (dB)
|
0.64
|
0.62
|
|
Total reflectivity level Value
R (dB)
|
-38.7
|
-39.0
|
Azimuth angle +20°-20° Directional pattern level value A (dB) 1010 Maximum level change value Δ (dB) 0.64 0.62 Total reflectivity level value R (dB) -38.7-39.0
It can be seen from the above test data that the reflectivity level of the tested small cylindrical microwave dark box measured by the above method at the selected frequency is better than the technical indicator of -30dB required by the design.
References:
[1] WANG Maoguang. Principle of Geometric Diffraction. Xi'an: Northwest Telecommunications Engineering Institute Press, 1985.
[2] Jiang Xianzuo. Antenna Principles. Beijing: Beijing University of Aeronautics and Astronautics Press, 1993.
[3] Mao Naihong. Antenna Measurement Handbook. Beijing: National Defense Industry Press, 1987.








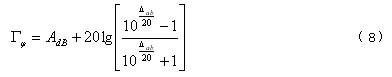





 Dual Radar: A Dual 4D Radar Multimodal Dataset for Autonomous Driving
Dual Radar: A Dual 4D Radar Multimodal Dataset for Autonomous Driving CVPR 2023 Paper Summary: Video: Low-Level Analysis, Motion, and Tracking
CVPR 2023 Paper Summary: Video: Low-Level Analysis, Motion, and Tracking















 京公网安备 11010802033920号
京公网安备 11010802033920号