Analysis of flux weakening control of permanent magnet synchronous motor based on voltage feedback

1 Introduction

There are two main ways to control the weakening magnetic field of permanent magnet synchronous motors, one is based on feedforward and the other is based on feedback. Feedforward weakening magnetic field control uses the precise parameter modeling of the motor to generate the current command when the motor is running. This method has a fast response speed, but the cost is high. The other is a method based on voltage feedback. The voltage feedback method uses the maximum allowable voltage of the inverter as the reference value, and the stator voltage set value output by the current loop as the feedback value to build a voltage loop to adjust the weakening magnetic current command. Although the voltage feedback method is not as fast as the feedforward method in response speed, it is not sensitive to motor parameters and is easy to deploy. The editor believes that there are two main reasons for the slow response speed of the voltage feedback method. One is that the parameters of the voltage loop controller are not suitable and the performance is not ideal; the other is that the voltage loop controller has overshoot and needs to reserve a large voltage margin. There have been literatures promoting solutions to these two problems. This article aims to share with readers the research results on the voltage loop in voltage feedback weakening magnetic field control in recent years. I hope that readers in need can conduct further exploration based on these literatures. Due to time and energy limitations, this article does not conduct a detailed and in-depth analysis. If you have any questions, please discuss in the Simo forum.

2 Voltage Weakening Control Principle

The basic principles of permanent magnet synchronous motor weakening control, including the concepts of current circle and voltage circle, can be found in the motor control book [1]. This article will not go into details. Only the block diagram of voltage feedback weakening control is briefly introduced. Figures 1 and 2 are block diagrams of two types of voltage feedback. Figure 1 shows the direct adjustment of ∆id using the voltage error. The obtained ∆id is superimposed on the id of the MTPA to form the current given value. Correspondingly, iq is adjusted according to the change of id and the constraint of the current circle [2].

Figure 1 Block diagram of voltage feedback weak magnetic control (ID)

Figure 2 shows the direct adjustment of the current vector angle. The speed loop outputs the current vector amplitude. The voltage loop outputs the current vector angle. The required current given value can be obtained by calculating the two.

Figure 2 Block diagram of voltage feedback field weakening control (current vector angle)

3 Voltage loop gain adaptation

One of the problems with the voltage feedback field weakening control method in Figures 1 and 2 is that the fixed-parameter PI controller cannot cope with the nonlinearity caused by the change of the small signal model at different operating points. This section will analyze the change of the small signal model at different operating points and introduce the gain linearization method [2].

The voltage equation of the permanent magnet synchronous motor is (1). Based on the voltage equation, we can calculate the amplitude of the stator voltage as (2).

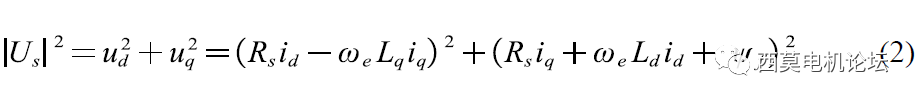
Since the angular velocity varies slowly relative to the current variable, the stator voltage amplitude can be considered as a constant component plus a variable component affected by the current.

During the field weakening process, the stator voltage amplitude always remains at the maximum, therefore, .

For the changing component of the stator voltage amplitude, it can be expressed as

Where X can be the current vector angle β or id, depending on the type of field weakening control variable.

Taking the current vector angle β as an example,

Using and , combining (1) and (2) we can get

According to (6), combined with the current operating point in the weak magnetic field region given in the 37th seminar, the voltage vector small signal model gain diagram can be obtained as shown in the figure.

Figure 3 Voltage loop small signal model gain corresponding to different current vector angles

It can be seen that at different current vector angles (operating points), the small signal model gain between the voltage vector and the system variables is quite different. This shows that the voltage loop itself has very strong nonlinear characteristics in the weak magnetic region. There are three system variables in Figure 3. Among them, the nonlinearity of id is the strongest. The nonlinearity of β and iq is slightly weaker. Of course, if a PI controller is used directly in the voltage loop, it is obviously difficult to cope with such strong nonlinearity. Therefore, a gain compensation unit can be added to the voltage loop to normalize the gain of the voltage loop, which is beneficial to the deployment of the PI controller. The expression for the gain has been given in equation (6). In fact, (6) can be simplified to the following form

Therefore, we can get the gain compensation value as

4 Transfer function of voltage loop

Figure 4: Block diagram of voltage feedback loop

Figure 4 is a block diagram of the voltage loop. The open-loop transfer function Gvol of the voltage loop can be derived from this block diagram. In Gvol, Ru is the stator voltage controller of the voltage loop. D is the system delay. us is the transfer function from the current vector angle to the stator voltage us. According to the control block diagram of the voltage loop, the open-loop transfer function of the voltage loop is

In the formula, the voltage loop regulator

System Latency

Transfer function from current vector angle to stator voltage

In the formula, the transfer function of the current regulator is

Transfer function of current loop

Electrical transfer function of permanent magnet synchronous motor

5 Simplification of voltage loop transfer function

In the previous section, we obtained the transfer function of the voltage loop, but this transfer function is very complex and cannot effectively guide the design of the voltage loop controller parameters. This section will introduce a simplified design method for the voltage loop [3,4].

For the adaptive gain of the voltage loop, we use the method in Section 2. However, the difference is that this method introduces the transfer function between the stator voltage amplitude and id, so the small signal gain between the stator voltage and id is

Since Ud is mainly affected by the disturbance of iq, the relationship between Ud and iq is replaced according to (19), and (20) is obtained

Here, we need to deal with the derivative between iq and id. When the torque is constant, the derivative between iq and id is

Therefore, combined with (18), we can get

Putting the gain in (22) into the loop, the voltage loop can be expressed as shown in Figure 5

Figure 5 Block diagram of voltage loop

In the figure, the gain linearization coefficient of the voltage loop is added. In this way, the gain from id to U at each operating point is normalized. Therefore, the gain from id to U in the figure cancels out with Knorm, and the open-loop transfer function of the voltage loop becomes

Wherein, the closed-loop transfer function of the current loop has been simplified under the assumption that the parameters of the current loop controller Ri are idealized, and α is the bandwidth of the current loop.

In this way, the remaining work is to configure the PI parameters of the voltage loop, Kvp and Kvi. Here, the zero-pole cancellation method is used to adjust the transfer function. From (23), we can get:

Then the open-loop transfer function of the voltage loop becomes

From (25), we can see that the bandwidth of the voltage loop is The last task is to determine the bandwidth of the voltage loop. Since the voltage loop needs to respond faster than the speed, but since the voltage loop controller outputs the given value of id, the response speed of the current loop should be slower than the voltage loop. In short, the bandwidth of the voltage loop needs to be between the bandwidth of the current loop and the bandwidth of the speed loop. Generally, the bandwidth of the voltage loop can be taken as half of the bandwidth of the current loop. More specifically, voltage margin and voltage drop are all factors that need to be considered when setting the bandwidth. These are analyzed in detail in the literature [3].

References

[1] SUL S K. Control of electric machine drive systems[M]. John Wiley & Sons, 2011.

[2] BOLOGNANI S,CALLIGARO S, PETRELLA R. Adaptive Flux-Weakening Controller for InteriorPermanent Magnet Synchronous Motor Drives[J/OL]. IEEE Journal of Emerging andSelected Topics in Power Electronics, 2014, 2(2): 236-248.

[3] BEDETTI N,CALLIGARO S, PETRELLA R. Analytical design and auto-tuning of adaptiveflux-weakening voltage regulation loop in IPMSM drives with accurate torqueregulation[C/OL]//2017 IEEE Energy Conversion Congress and Exposition (ECCE).2017 : 5884-5891.

[4] JACOB J,CALLIGARO S, BOTTESI O, et al. Design Criteria for Flux-Weakening ControlBandwidth and Voltage Margin in IPMSM Drives Considering TransientConditions[C/OL]//2019 IEEE Energy Conversion Congress and Exposition (ECCE).2019: 5667-5674.